The Checker Maven
The World's Most Widely Read Checkers and Draughts Publication
Bob Newell, Editor-in-Chief
Published every Saturday morning in Honolulu, Hawai`i
Noticing missing images? An explanation is here.
Gibson

Yes, that's a Gibson Les Paul guitar, made by the Gibson company. The famous guitar manufacturer was founded by one Orville Gibson in 1902, and revolutionized guitar design with an arch-top model, based on the design of the violin.
Our current Checker School series is featuring "snappy" problems by G. M. Gibson. We don't know anything about this gentleman; was he a contemporary of Orville, or a relative or descendant? Did Orville play checkers himself?
These questions will remain unanswered for the moment. But we're happy to present another of Mr. Gibson's "snappy" (or should we, to accord with our metaphor, call it "twangy"?) problems.
WHITE

BLACK
B:WK7,20,K25:B4,K14,K15.
Forces are even, although Black, with centralized kings, may have more mobility. Can you snap out a win from this one? Pick away at it and then strum your mouse on Read More to see the solution.![]()
Clever, Clever

Clever, Montana, is a real place, a fast-growing small town of about 3,000 with the motto "It just gets better every day." The town is well over 100 years old and it's pretty likely to have some sort of checker playing history. Do folks still play checkers in Clever? We emailed a town official but we never got a reply, so we can't say for sure.
There's certainly a place for cleverness in the game of checkers, and today's problem, a "not quite" speed problem, relies on a clever first move.
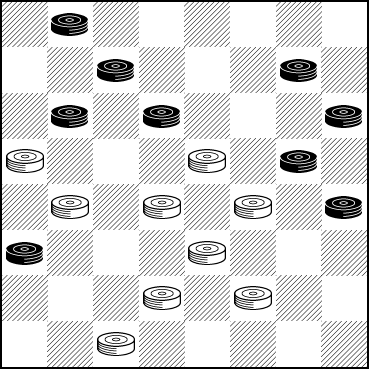
WHITE
White to Play and Draw
W:W13,15,17,18,19,23,26,27,30:B1,6,8,9,10,12,16,20,21.
Are you clever enough to solve it? We think you are, but when you're done, the clever thing to do would be to click on Read More to check your solution.![]()
Labor Day 2018

Labor Day is another one of our favorite holidays, for it gives us the chance to recognize the contributions of the average guy and gal, the working woman and working man who are the backbone of America's prosperity. Every day these folks put in an honest day's work and ask no more than the chance to get ahead a little, to take care of their families, and to do their part in building a society that will offer something to everyone.
As we've pointed out in previous years, many checkerists were and are "ordinary" people who in fact are quite extraordinary. Checker champions have been baseball players and steel workers and just about anything you can think of. Checkers knows no boundary of class or status, and we can't help but feel good about that.
We turn as always to Tom Wiswell, with something he called Fantastic. He could have easily been describing himself, but of course Mr. Wiswell was modest and humble as are most of the truly great.
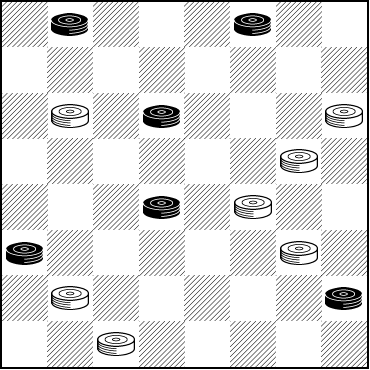
WHITE
White to Play and Win?
W:W9,12,16,19,24,25,30:B1,3,10,18,21,28.
Now, we're going to do something different this week. We'll give you the solution up front. Here is what Mr. Wiswell published quite some years ago.
| 1. | ... | 19-15 |
| 2. | 10x19 | 24x15 |
| 3. | 28-32 | 15-10 |
| 4. | 32-27 | 9-6 |
| 5. | 18-23 | 6-2 |
| 6. | 23-26 | 30x23 |
| 7. | 27x18 | 2-7 |
| 8. | 21x30 | 12-8 |
| 9. | 3x19 | 10-6 |
Mr. Wiswell's solution ends here. Continue:
| 10. | 1x10 | 7x16 |
White Wins with the opposition.
But the thing is that Mr. Wiswell, very uncharacteristically, was wrong. The position is actually a draw. So what you ask you to labor away at is this: Correct the play above and show how Black can draw. You've got your work cut out for you, and the task is not easy. But, as the saying goes, that's why they call it "work." When you're ready, work your mouse over to Read More to see the annotated and corrected play.![]()
Mr. Scissors

It looks like something straight out of a horror movie; the evil villain (let's call him Mr. Scissors) threatens our heroine with a horrible fate. But of course, we know that the hero will arrive to save the day ... or will he?
The villain in the old movie above certainly isn't regular contributor and master problemist Ed Atkinson, who sent us today's problem, which he of course calls Mr. Scissors.
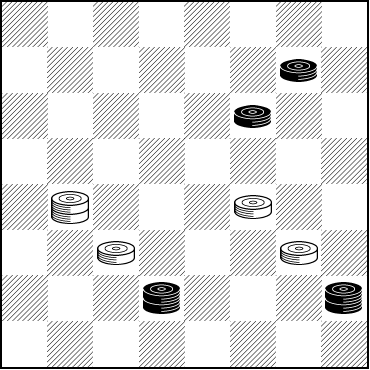
WHITE
White to Play and Win
W:WK17,19,22,24:B8,11,K26,K28.
Once you find the right first move, the problem isn't all that hard. Can you cut it? Snip away and save the day, then cut your mouse over to Read More to see the cutting-edge solution.![]()
Popularity
Popularity is fleeting. One day you're in ...

... the next day, you're out.

Our game of checkers, too, has gone through such cycles.
In 1908, a match was played for the championship of Essex County, Massachusetts. One can only imagine with wonder at the popularity of checkers 110 years ago, at a level such that even county championships were vigorously contested.
The match was played between C. O. Mayberry, who was champion of the city of Lynn (yes, there were municipal champions as well), and Frank L. McClellan, the Captain of the Lynn Checker Club (in additional to the local club, the Lynn newspaper published a checker column). In the position below, Mr. Mayberry played Black and Mr. McClellan, White. The setting was originally featured in Teetzel's Canadian Checker Player. Mr. Teetzel opines that Mr. Mayberry must have thought he was going to win, but it was not to be, as Mr. McClellan found a clever draw.

WHITE
White to Play and Draw
W:W9,16,18,20,26,30:B1,3,7,11,21,K29.
Checkers is, sadly, far less popular today, but that doesn't mean we shouldn't continue to enjoy the game. Will the problem above prove "popular" with you? We think so, and after you solve it, we're sure you'll enjoy clicking on Read More to check your solution.![]()
Another Snappy One

Wow, we'd hate to be on the receiving end of whatever is going on in the photo above; that lady is really snapping at someone. We can only hope it all gets worked out peacefully.
We're continuing our Checker School series with another "snappy" problem posed to our friend Nemo by his mentor, Skittle, both of whom appear in Checker Board Strategy by Andrew J. Banks, a self-described "checker philosopher." The problem itself is attributed to G. M. Gibson.
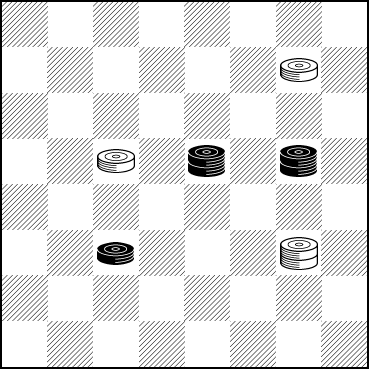
BLACK
Black to Play and Win
B:WK9,19,25:B11,K17,K18.
Black has two kings and a man to White's one king and two men, and has one other obvious advantage. Do you see it? Do you see how White might defend, and how Black might still overcome that defense?
Don't snap at us; we're just trying to provide you with interesting material! And when you find the solution, you'll surely snap to attention! Clicking on Read More will let you check your work and review our extensive notes.![]()
Sort Of

"Sort of" is a common two-word phrase in English. We're "sort of" tired or hungry. We "sort of" need to do homework, laundry, yard work, etc. And the best example of all: We're "sort of" interested in doing something or going somewhere.
We hope that all of us are more than "sort of" interested in checkers, though; and if we are, you'll find this "sort of" speed problem (pun intended), provided by regular contributors Lloyd and Josh Gordon, to be a good one.

WHITE
White to Play and Draw
W:W14,15,18,19,24,27,28,30:B1,3,6,7,8,11,20,22.
Why is this a "sort of" speed problem? The initial sequence is easy to find, but the follow-up play is a bit more complex, though certainly below the expert range. So don't "sort of" solve it; do it all the way, after which clicking on Read More will more than "sort of" show you the solution and explanatory notes.![]()
Checker School: Second Session

We've completed our first session of Checker School, which was a tour through Ben Boland's Famous Positions in the Game of Checkers. For our next session, we'll turn (at least at first) to an unusual book published by Andrew J. Banks in 1945, called Checker Board Strategy. Mr. Banks, who lived in Washington, D.C., evidently self-published his work.
The book is written in an entertaining style and features a number of fascinating fictional players. We'll get to meet them as the months roll by. Mr. Banks starts out with the rules of checkers (compiled by none other than William Ryan) and then continues with a brief games section that illustrates the basic openings. Next is a section he calls Snappy Problems (Gems) Today, we'll look at the first one and along the way make the acquaintance of Nemo and Skittle.
Nemo had been studying the foregoing games (in the Games Section--Ed.) when Skittle exclaimed, "You are learning checkers the hard way. You are like a tourist I saw in the State of Maine; he stoped a native and inquired 'How far is it to Portland?'"
"How far was it?" Nemo asked.
"The way the tourist was headed it was about 25,000 miles. The native told him that if he would turn around and go the other way it would be only about two."
"You be my guide; show me the quick way to learn the game," said Nemo.
"By solving problems you will be learning checkers the quick way."
Champion player Alex Moiseyev flatly states that beginners should not touch opening books until they have played a large number of games; many other checker greats stress the value of solving problems. So, the first "gem" or "snappy" problem proposed by Mr. Banks is this one, by G. M. Gibson.
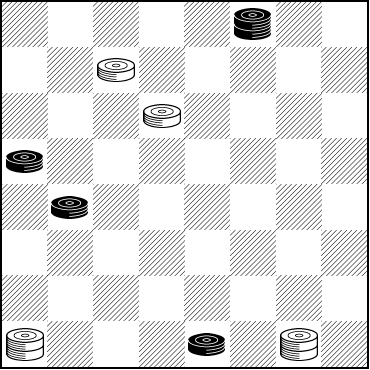
BLACK
Black to Play and Win
B:W27,23,K4,K1:BK30,20,16,2.
Can you solve the problem proposed to Nemo by our new friend Skittle? Make it snappy! Solve it and then snap your mouse on Read More to check your solution.![]()
Bastille Day
July 14 is the national holiday of France, generally known as Bastille Day. Popular wisdom is that the holiday commemorates the capture of the Bastille on July 14, 1789, during the revolution which brought down the French monarchy.
But we learn something every day. When we consulted the official French government website, we were told:
«Si le 14 juillet est généralement associé à la prise de la Bastille en 1789, c'est dans les faits le 14 juillet 1790, la fête de la Fédération, qui est officiellement commémoré en France.»
We'll bet you didn't know that, either.
Now, it seems that in honor of Bastille Day, we should present a problem by a French problemist, but in the 8x8 Anglo-American literature, we didn't find one that we could specifically attribute. However, Jean-Bernard Alemanni, in his excellent book "Les jeux de dames dans le monde" does present a couple of relevant demonstration positions. While these are surely not original compositions, the one we show below makes for an easy practice exercise.
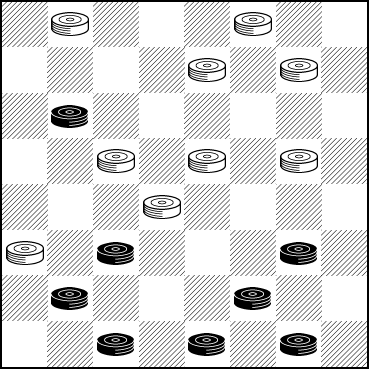
BLACK
Black to Play and Win
B:W12,15,17,18,19,25,26,30,32:B1,2,3,6,8,9,11,24.
Vite, vite! Trouvez la solution, and when you're done cliquez votre souris sur Read More to check your answer.![]()
Friday the 13th

Paraskevidekatriaphobia. It's what fear of Friday the 13th is called in scientific language (actually, it derives primarily from Greek), and many people won't even go out of doors on that date. There are many ideas as to its origin, but it's a fear not universally held. In fact, in Cantonese speaking areas such as Hong Kong, 13 is considered a lucky, not unlucky, number--- so presumably Friday the 13th would be a lucky day.
Friday the 13th is coming up in the week following initial publication of this column; how will you greet the day?
Unsurprisingly, we suggest greeting it with a thematically relevant checker problem such as the one below, composed by grandmaster problemist Ed Atkinson.
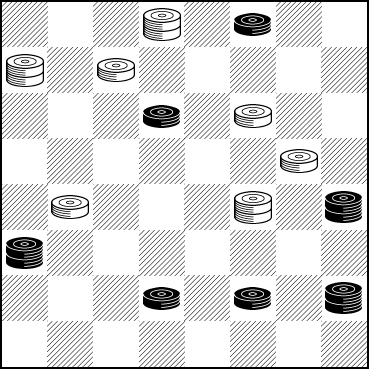
WHITE
White to Play and Win
W:WK2,K5,6,11,16,17,K19:B3,10,K20,K21,26,27,K28.
Will you be lucky or unlucky, or do you believe it's all a matter of skill? Try your luck, and then luck out by clicking on Read More to see the incredible solution.![]()
The Checker Maven is produced at editorial offices in Honolulu, Hawai`i, as a completely non-commercial public service from which no profit is obtained or sought. Original material is Copyright © 2004-2025 Avi Gobbler Publishing. Other material is public domain, as attributed, or licensed under Creative Commons. Information presented on this site is offered as-is, at no cost, and bears no express or implied warranty as to accuracy or usability. You agree that you use such information entirely at your own risk. No liabilities of any kind under any legal theory whatsoever are accepted. The Checker Maven is dedicated to the memory of Mr. Bob Newell, Sr.

