The Checker Maven
The World's Most Widely Read Checkers and Draughts Publication
Bob Newell, Editor-in-Chief
Published every Saturday morning in Honolulu, Hawai`i
Noticing missing images? An explanation is here.
4th of July Special

When this column appears we'll be just a few days short of the 4th of July, America's birthday, and a holiday that The Checker Maven celebrates every year; for as we always say, we are unabashed patriots, proud to honor our nation on Independence Day.
And--- as we do every year--- we turn to player, problemist and patriot Tom Wiswell, with another of his studies. He calls this one Harmony.
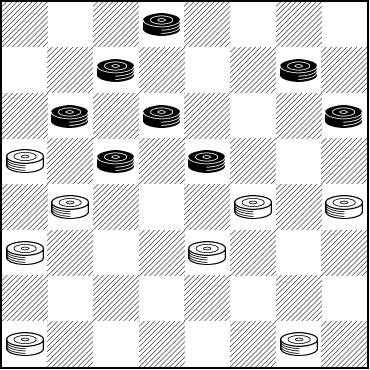
WHITE
White to Play and Draw
W:W13,17,19,20,21,23,29,32:B2,6,8,9,10,12,14,15.
Mr. Wiswell suggests that the pieces should work together to sing a harmonious tune. We agree; can you play the correct melody, without any flat notes? Try to solve it, then let your mouse sing (a patriotic tune) on Read More to see the solution and notes.![]()
Novice Lesson

Yes, there is such a place. It's a small town in Texas, population not much over 100. How many of those residents, do you suppose, are checker players?
We can't really say, but we can say that in honor of the release of the print edition of Checkers for the Novice, today we're presenting something vital, yet which actually won't be very easy for novices. Experts, of course, should have no problem --- right?
3 kings vs. 2 kings seems like it ought to be an easy win, yet it in fact baffles many a player, even some players with a fair amount of experience with our game. Let's look at an example, in two different ways.
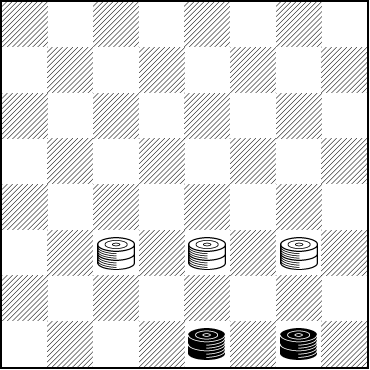
WHITE
Either to Play, White to Win
W:WK22,K23,K24:BK31,K32.
First, try this with White to play. How does White win it? If you're a top-level player, you'll see how to do it in just a few seconds --- right?
How about if Black plays first? Can you win with White? It's a little harder, but again an expert should solve it fairly quickly --- right?
We're teasing a little. Even most experts have to stop and think. 3 vs. 2 endings aren't easy, but they come up all the time and knowing how to win them is essential. Take as much time as you like and then click on Read More to see the solutions.![]()
Strokes in June

In order to avoid reuse of the "golf stroke" pun, we've gone with a tennis stroke instead. The point is that winter in North America seemed especially tough this year (or at least so we hear[1]), and we'd bet that many of you are happy to be able to get out and make a few strokes on the tennis court or golf course.
But, as a checker fan, surely you'll come back in at the end of the day, and, perhaps after dinner, want to take on a checker stroke, one of those mind-bending fantasy problems that may not be practical but certainly provide great visualization practice. With that in mind, here's one that we would not call easy.
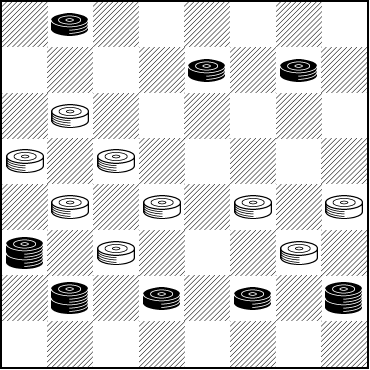
WHITE
White to Play and Win
W:W24,22,20,19,18,17,14,13,9:BK28,27,26,K25,K21,8,7,1.
Take a swing at this rather entertaining position, then swing your mouse over to Read More to see the solution.![]()
[1] This year we did not have snow in Honolulu(!) although the temperature did get as cold as 62F/17C.
[Read More]The Engineer

Although your editor's degrees are in engineering, he's definitely not the kind of engineer shown above. Those engineers certainly earn a very nice living, and we suspect that quite a number of them play checkers while on the road.
Today's little problem was published decades ago by someone who simply called himself "The Engineer." We have no further information on who he might have been. Did he design bridges? Refine oil? Drive a train? Perhaps someone out there on the internet might know, but for now it remains an intriguing mystery.
His checker problem, though, won't stay a mystery for long.

BLACK
Black to Play, What Result?
B:WK31,28,27,19:B20,18,12,K10.
Despite the sometimes unfair (and annoying!) "what result" terms, the problem is fairly easy if you take an engineering approach and do a little organized analysis. No slide rules required, just some orderly checker thinking. Do your calculations and then click on Read More to see the solution.![]()
A Bit of a Break

Sometimes, it all gets to be a little too much, and we need a break. We're not sure what the poor fellow in the picture above is working on; could he perhaps be doing a manual transcription of the 10-piece endgame database?
Today we have a speed problem, provided by regular contributors Lloyd and Josh Gordon, that will give you a break in checker terms: it's definitely on the easy side. While some may disagree, we believe that easy problems have a clear purpose: to improve both sight-solving skills and speed of analysis.

BLACK
Black to Play and Win
B:W32,30,19,K15,K6:BK26,24,23,21,12,7.
See how quickly you can solve this one, and then break over to Read More to verify your solution.![]()
A 'Maid of the Mill' Surprise

Above, a group of Morris Dancers are performing Maid of the Mill at a festival in Newcastle. Maid of the Mill spans many genres: dance, art, literature ... and draughts.
In our ongoing serialized story, Three Move Opening: A Checker Romance, our protagonist made an unfortunately timed reference to the Maid of the Mill opening. Today, we'd like to offer something quite substantial in the play of that opening.
The following is based on the 15th game of the 2014 World Championship Go As You Please match between Sergio Scarpetta (Black) and Ron King (White). The game, as played, ended in a draw, but there was an interesting winning possibility for Black.
| 1. | 11-15 | 22-17 |
| 2. | 8-11 | 17-13 |
| 3. | 15-18 |
Forms Maid of the Mill.
| 3. | ... | 23x14 |
| 4. | 9x18 | 21-17 |
| 5. | 10-15 | 25-21 |
24-20 is definitely better, but it isn't clear that this move loses.
| 6. | 4-8 | 26-23 |
| 7. | 18-22 | 24-19 |
| 8. | 15x24 | 28x19 |
| 9. | 11-16 |
Back into the KingsRow opening book with a pull for Black, but not clearly a win.
| 9. | ... | 27-24 |
| 10. | 8-11 | 24-20 |
| 11. | 7-10 | 32-28 |
| 12. | 3-7 | 28-24 |
| 13. | 11-15 | 20x11 |
| 14. | 7x16 | 24-20 |
23-18 also draws.
| 15. | 15x24 | 20x11 |
| 16. | 12-16 | 11-8 |
Loses; 23-18 should draw.
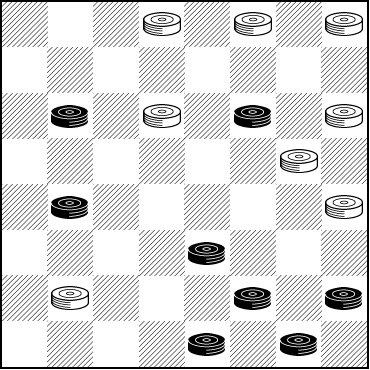
BLACK
Black to Play and Win
B:W31,30,29,23,21,17,13,8:B24,22,16,10,6,5,2,1.
Mr. Scarpetta didn't find the win. Can you? We realize that's a lot to ask, and in fact it's a very difficult problem, but the solution is as surprising as it's good, and we think that any effort you put into this problem will be amply repaid. So do try it, and then click on Read More to see the answer.![]()
A Possible Misstep

Snow can still come in April in many parts of North America, and if it does, you had best be careful and not suffer the type of mishap that of the unfortunate lady above.
This month's speed problem doesn't have a time limit and doesn't use the Javascript clock. Now, it's not a particularly hard problem, it's just that ... well, there's a way to misstep and slip, even if it doesn't involve snow and ice, and then things get a little more complicated.
Perhaps it's simplest to show you the diagram and let you find out for yourself.
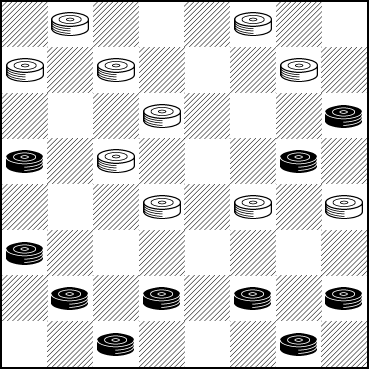
BLACK
Black to Play and Win
B:W32,30,28,27,25,23,19,15,14,13:B21,20,17,12,8,7,6,5,3,1.
Watch your step, and solve this step by step. The next step? Clicking on Read More to check your solution.![]()
Thinking It Over

The sculpture above is found near the Pioneer Museum in Colorado Springs, Colorado, a city we've visited a few times. We don't know what the young lad is musing upon; could that be a book of checker problems next to him?
Whatever he's contemplating, it's unlikely that it's today's checker problem, another fine entry sent to us by master composer Ed Atkinson. He calls it Thinking It Over. Let's let him describe it in his own words.
"Here is the problem. It is only the unusual setting and the first few moves that are original. The resulting end game can be traced back through the centuries to the very first problem published in English, a 262 year time line.
The references can be found in Boland's Border Classics, page 59 and Famous Positions, page 8. Closely related material was published by several in the mid 19th century."
BLACK
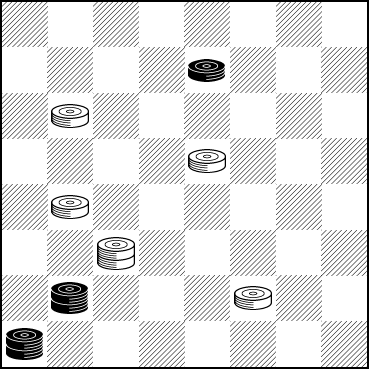
WHITE
White to Play and Win
W:W27,K22,17,15,9:BK29,K25,7.
What's this? White is up two pieces, so where's the problem? But it won't take you long to realize that White's big advantage is greatly at risk, and getting the full score is anything but easy.
Okay, you know what we're going to say: think it over, and find the winning moves. As often is the case, the key is to find the right first move. After you've given this enough thought, think about clicking on Read More to see Ed's solution and notes.![]()
Find Both Solutions
You probably learned in basic math classes that any quadratic equation has dual solutions, though they may not be unique, and when solving such equations, you were surely asked to find both solutions.
But as we've noted before, in checker problem competitions, "dual" solutions are frowned upon; a composition should have a but a single path to correctness. But with our speed problems, and with a mind to improving over the board visualization skills, sometimes a problem with a "dual" can be of value --- if you can find both solutions.
The following problem was sent to us by regular contributors Lloyd and Josh Gordon of Toronto, who developed it in conjunction with noted contemporary problemist Bill Salot. It has a dual solution.
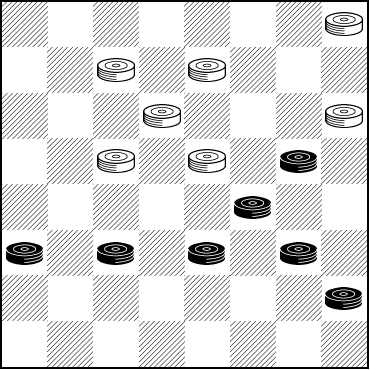
BLACK
Black to Play and Win
B:W18,19,21,23,26,27,29:B5,9,10,11,12,14,17.
Can you find both paths to victory? You'll get half credit for finding one of them, but full credit only if you work out both. The challenge is fair in that one solution is not simply a variant of the other.
Give this at least a "couple" of tries, and then click on Read More to see how you've done.![]()
Patterns

Ed Atkinson, of Harrisburg, Pennsylvania, is well-known in the checker community as a top player and skilled problemist. He is also, we're proud to say, a regular Checker Maven reader and occasional correspondent.
Ed offered us an original problem for publication and as you might expect, it's a dandy. He calls it Patterns, and you'll see why if you find the winning moves.
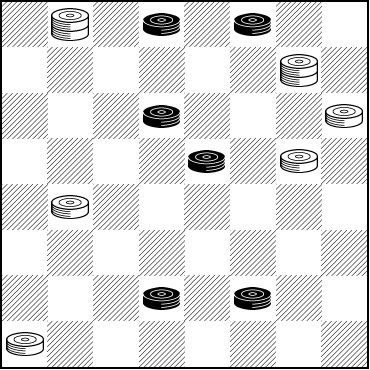
WHITE
White to Play and Win
W:W12,16,17,29,K1,K8:B2,3,10,15,26,27.
You'd think White would have an easy win here; after all, he's got two kings and Black has none. But White looks a bit crowded around Black's single corner, and the win isn't at all easy to find. This is a top-notch, difficult problem with a rewarding solution. Can you find the pattern?
See what you can arrange, and then click on Read More to check your work.![]()
The Checker Maven is produced at editorial offices in Honolulu, Hawai`i, as a completely non-commercial public service from which no profit is obtained or sought. Original material is Copyright © 2004-2025 Avi Gobbler Publishing. Other material is public domain, as attributed, or licensed under Creative Commons. Information presented on this site is offered as-is, at no cost, and bears no express or implied warranty as to accuracy or usability. You agree that you use such information entirely at your own risk. No liabilities of any kind under any legal theory whatsoever are accepted. The Checker Maven is dedicated to the memory of Mr. Bob Newell, Sr.

