Yin and Yang. Opposites that are bound together as a whole. This ancient Chinese philosophy encapsulates the concept of dualism, wherein seeming opposites can actually be interconnected and interdependent, the one giving rise to the other. We'll leave it to you to explore the ideas of dialectical monism and how Yin and Yang are reflected in Taoist, Confucianist, and other philosophical realizations. It's a deep topic indeed and could form the basis for a lifetime of study.

Alex Moiseyev

Champion checkerist Alex Moiseyev has created a checker problem which he says embodies concepts of Yin and Yang. It's an amazing problem, itself deep and difficult. In fact, master problem composer Brian Hinkle has this to say about it, and it is through Brian's auspices that The Checker Maven is presenting the problem in its first-ever public appearance.

This 9x10 bridge with five Kings called 'Yin And Yang' composed by Alex Moiseyev is one of best checker problems I have ever seen. Master checker players may find it challenging to solve. I enjoyed the pretty solution so much that I looked at it about four times a day for a week!

BLACK

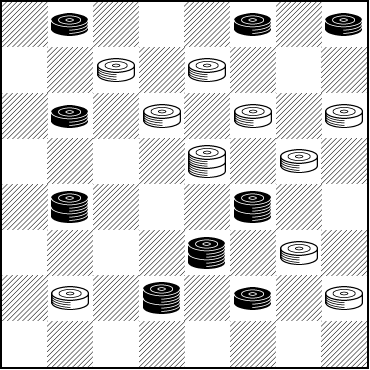
WHITE
White to Play and Win

W:W6,7,10,11,12,K15,16,24,25,28:B1,3,4,9,K17,K19,K23,K26,27

No, it's not easy, but yes, it's really something and well worth taking the time to study and appreciate. See how far you can go with it. Search for its echoes of dialectical monism. Discover its inherent, interconnected dualism. Finally, click on Read More for the solution, notes, and some background on the problem's genesis.

Solution

Problem and solution by Alex Moiseyev. Unattributed notes by Brian Hinkle.

12-8*---A,B,C 3x12---D 15-18 23x14 24x15 12x19 11-8 4x18 7-2 14x7 2x11 1x10 11-15 White Wins---E

A---This remarkable move gives Black two ways to jump, 3x12 (yin) as shown in the trunk or 19x12 (yang) as shown in Note D.

B---25-21?---G 17-22*---H 24-20 19-24 28x19. Black is two men down but the massive binding and lockdown of the White pieces prevents White from promoting and freeing its pieces, and eventually the game could end up in a see-saw draw---Alex Moiseyev.

C---24-20? 27-31 15x24 17-21 and with the bridge and Black Kings chasing, Black will draw a man down.

D---19x12 24-19 23x16 7-2 16x14 15-19 4x11 19-24 1x10 24x8 (yang).

E---The White King on 15 slices through the Black pieces like a hot knife through butter. Black has 17 ways to try but White wins against everything, for example 17-22---F 15-31 22-29 31-13 White Wins (yin).

F---Or if Black moves the other King 26-22, then White jumps in the opposite direction with 15x13 22x29 13x31 White Wins. Please note the symmetry.

G---Composers should note that having a King on 26 (instead of a man) prevents the first move 25-21 from forming a dual solution.

H---If 17-13? 24-20 White wins.

History of this Problem (by Brian Hinkle)

Alex Moiseyev is a recognized Grandmaster composer and has composed thousands of problems in 10x10 International Draughts, winning the World Composing Championship in 2016. Knowing this, I asked Alex, "How can you compose thousands of 10x10 problems but not compose anything in the style in which you were world champion?" Presented with this question and with the postponement of his world title match with Sergio Scarpetta, Alex started composing for English checkers and as of August 2020 has composed more than 10 problems. I sent Alex a 7x3 four-man down White Win setting, which I composed, with the challenge "Let’s see how high you can fly!" About eight hours later, he emailed me back the problem above, which he named "Yin and Yang." Alex graciously wanted to equally credit both of us but in fairness the serious creative talent was demonstrated by Alex, who converted my problem into "Yin and Yang."

The Checker Maven editorial staff hopes you enjoyed this unique checker problem. We extend our thanks to both Brian and Alex for the opportunity to publish it. And perhaps now you'll agree that even checkers reflects the principle of dialectical monism that "for the dialectical monist, reality is ultimately one but can only be experienced in terms of division."