Here's a problem attributed to O. H. Richmond, and published a little over 50 years back by Tom Wiswell. He called it "Black Beauty":

The White forces in the Black double corner are really boxed in, but Black has to find a way to make that into a win.

Now, you'll surely find that this is a neat problem and a "real twister" as Wiswell would say. So solve it, check your solution with the animation here, and then click on Read More to find out what the computer has to say about this problem. It won't be what you think--- or will it?

Did you like that one? OK then, let's go back to that key position:

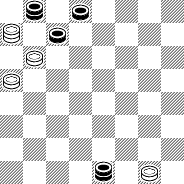
At this point Wiswell gave 9-6 as shown in the animation; as explained there, this is the only chance for White to break the blockade. Any other move and Black scoots off with 10-15 and now the lone Black king on 1 holds three Whites at bay while Black goes on to crown and eats up the other White king at his leisure.

So if you give this position to a strong computer program, you'd see White play 9-6, right?

No way.

The computer would play something like 8-12!

But.... doesn't the computer understand? Well, in fact, it understands very well that 9-6, although it breaks up the impasse in the Black double corner, leads to a flashy loss a few moves later. So, realizing that the game is lost anyway, the computer will play whatever moves it thinks will drag the game on longer. 8-12, despite being an obvious loss, will take longer to lose than an alternative such as 9-6. So 8-12 it shall be.

In fact, this is often an issue when using the computer to review endgame situations, particularly composed problems, and especially clever ones! The computer, seeing more than most of us, will sometimes play in a manner that appears unnatural--- seemingly taking an obvious loss, or pitching a piece, or something that to us appears strange--- because doing that will make the game last longer, no matter that it's lost in all variations. A longer loss is considered better than a shorter loss.

Although I am a big fan of the computer as a teacher and study partner, as I've written about extensively on this web site, there are times to be cautious and to take the computer's play with a grain of salt. The play may be technically correct in every way, but it may not be the type of play you wish to imitate in other situations that may seem similar, but may have subtle differences. You don't have a multi-gigabyte database stored in your brain; the computer does.