The Checker Maven
Jump to navigationComplex Interchange

Does the word "interchange" call to mind the kind of hopelessly complicated tangle of roadways depicted above? We're not sure if this photo is real or satire, but please remind us to seek an alternative route.
In checkers, "interchange" can have different meanings, the most common ones probably referring to an exchange of pieces or an exchange of positions.
Today, we'll present a study that takes the idea to its ultimate conclusion. This is not a typical checker problem, but it has a great deal of didactic value. The exact origin of this problem is unknown, but it's been around for a while.
The problem is to go from the start position
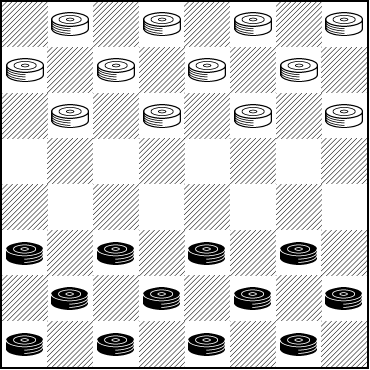
BLACK
Starting Position
B:W32,31,30,29,28,27,26,25,24,23,22,21:B12,11,10,9,8,7,6,5,4,3,2,1.
to the following fully interchanged position.
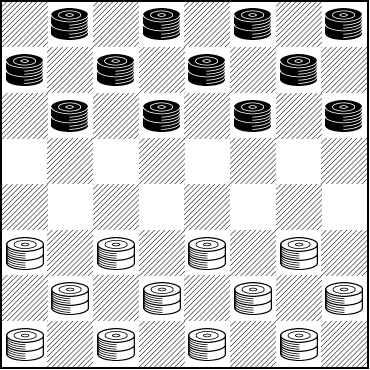
BLACK
Ending Position
B:WK12,K11,K10,K9,K8,K7,K6,K5,K4,K3,K2,K1:BK32,K31,K30,K29,K28,K27,K26,K25,K24,K23,K22,K21.
Of course, this has to be done completely with legal moves (e.g., all forced captures will have to be avoided).
Now, we won't say it's easy or short (it's neither), but a methodical, thoughtful approach will yield results. This is a great exercise in planning and visualizing, and we believe it will aid in the development of over-the-board skills. And in the process, you'll certainly learn something about mobility, traffic jams, and clearing a path.
Can you untangle this one, or will you loop around in your quest for a solution? It's worth your time and effort, but when you want to get out of the traffic, just click on Read More to see an animated solution.![]()
Solution
You're probably best off viewing the animation found here. But for the sake of completeness, the full solution is shown below in list form.
We don't claim this is the only solution, and certainly don't claim it's the shortest. But it is one way of working it out.
We hope you enjoyed today's excursion into something quite a bit out of the ordinary.
| 1. | 12-16 | 21-17 |
| 2. | 16-20 | 17-13 |
| 3. | 9-14 | 24-19 |
| 4. | 14-17 | 19-16 |
| 5. | 17-21 | 16-12 |
| 6. | 5-9 | 28-24 |
| 7. | 9-14 | 24-19 |
| 8. | 14-17 | 19-16 |
| 9. | 11-15 | 23-18 |
| 10. | 15-19 | 18-14 |
| 11. | 19-24 | 14-9 |
| 12. | 24-28 | 9-5 |
| 13. | 8-11 | 27-23 |
| 14. | 11-15 | 23-18 |
| 15. | 15-19 | 18-14 |
| 16. | 19-24 | 14-9 |
| 17. | 4-8 | 32-27 |
| 18. | 8-11 | 27-23 |
| 19. | 11-15 | 23-18 |
| 20. | 15-19 | 18-14 |
| 21. | 28-32 | 22-18 |
| 22. | 24-28 | 18-15 |
| 23. | 19-24 | 15-11 |
| 24. | 10-15 | 11-8 |
| 25. | 15-19 | 8-4 |
| 26. | 6-10 | 25-22 |
| 27. | 1-6 | 22-18 |
| 28. | 32-27 | 18-15 |
| 29. | 27-23 | 15-11 |
| 30. | 28-32 | 29-25 |
| 31. | 32-27 | 25-22 |
| 32. | 27-32 | 22-18 |
| 33. | 32-27 | 18-15 |
| 34. | 27-32 | 11-8 |
| 35. | 32-27 | 15-11 |
| 36. | 10-15 | 5-1 |
| 37. | 6-10 | 1-5 |
| 38. | 15-18 | 5-1 |
| 39. | 10-15 | 1-5 |
| 40. | 18-22 | 5-1 |
| 41. | 22-25 | 9-5 |
| 42. | 15-18 | 14-9 |
| 43. | 18-22 | 1-6 |
| 44. | 25-29 | 6-1 |
| 45. | 23-18 | 1-6 |
| 46. | 22-25 | 6-1 |
| 47. | 18-22 | 1-6 |
| 48. | 27-32 | 6-1 |
| 49. | 24-28 | 1-6 |
| 50. | 19-24 | 6-1 |
| 51. | 7-10 | 26-23 |
| 52. | 10-14 | 23-19 |
| 53. | 3-7 | 30-26 |
| 54. | 7-10 | 26-23 |
| 55. | 25-30 | 8-3 |
| 56. | 21-25 | 12-8 |
| 57. | 17-21 | 16-12 |
| 58. | 14-17 | 19-16 |
| 59. | 10-14 | 23-19 |
| 60. | 32-27 | 1-6 |
| 61. | 27-23 | 6-10 |
| 62. | 23-26 | 10-7 |
| 63. | 28-32 | 5-1 |
| 64. | 32-27 | 1-6 |
| 65. | 27-23 | 6-10 |
| 66. | 24-28 | 9-5 |
| 67. | 28-32 | 5-1 |
| 68. | 20-24 | 13-9 |
| 69. | 24-28 | 9-5 |
| 70. | 2-6 | 10-15 |
| 71. | 6-9 | 31-27 |
| 72. | 23-18 | 27-24 |
| 73. | 9-13 | 24-20 |
| 74. | 18-23 | 15-10 |
| 75. | 14-18 | 19-15 |
| 76. | 23-27 | 10-6 |
| 77. | 18-23 | 15-10 |
| 78. | 27-24 | 6-9 |
| 79. | 26-31 | 7-2 |
| 80. | 31-27 | 2-6 |
| 81. | 22-26 | 11-7 |
| 82. | 26-31 | 7-2 |
| 83. | 17-22 | 16-11 |
| 84. | 22-26 | 11-7 |
| 85. | 13-17 | 20-16 |
| 86. | 17-22 | 16-11 |
| 87. | 24-20 | 9-13 |
| 88. | 27-24 | 6-9 |
| 89. | 32-27 | 1-6 |
| 90. | 28-32 | 5-1 |
| 91. | 24-28 | 9-14 |
| 92. | 27-24 | 6-9 |
| 93. | 31-27 | 1-5 |
| 94. | 26-31 | 10-6 |
| 95. | 23-26 | 6-1 |
| 96. | 27-23 | 2-6 |
| 97. | 31-27 | 7-2 |
| 98. | 26-31 | 3-7 |
| 99. | 30-26 | 8-3 |
| 100. | 25-30 | 7-10 |
| 101. | 23-19 | 3-7 |
| 102. | 26-23 | 12-8 |
| 103. | 30-26 | 8-3 |
| 104. | 22-25 | 4-8 |
| 105. | 25-30 | 8-12 |
| 106. | 21-25 | 11-8 |
| 107. | 26-22 | 8-4 |
| 108. | 30-26 | 3-8 |
| 109. | 25-30 | 7-3 |
| 110. | 30-25 | 10-7 |
| 111. | 25-21 | 7-11 |
| 112. | 26-30 | 2-7 |
| 113. | 22-25 | 6-2 |
| 114. | 23-26 | 9-6 |
| 115. | 26-22 | 14-10 |
| 116. | 19-23 | 13-9 |
| 117. | 23-26 | 9-13 |
| 118. | 27-23 | 13-9 |
| 119. | 24-27 | 9-13 |
| 120. | 20-24 | 13-9 |
You can email the Webmaster with comments on this article.
